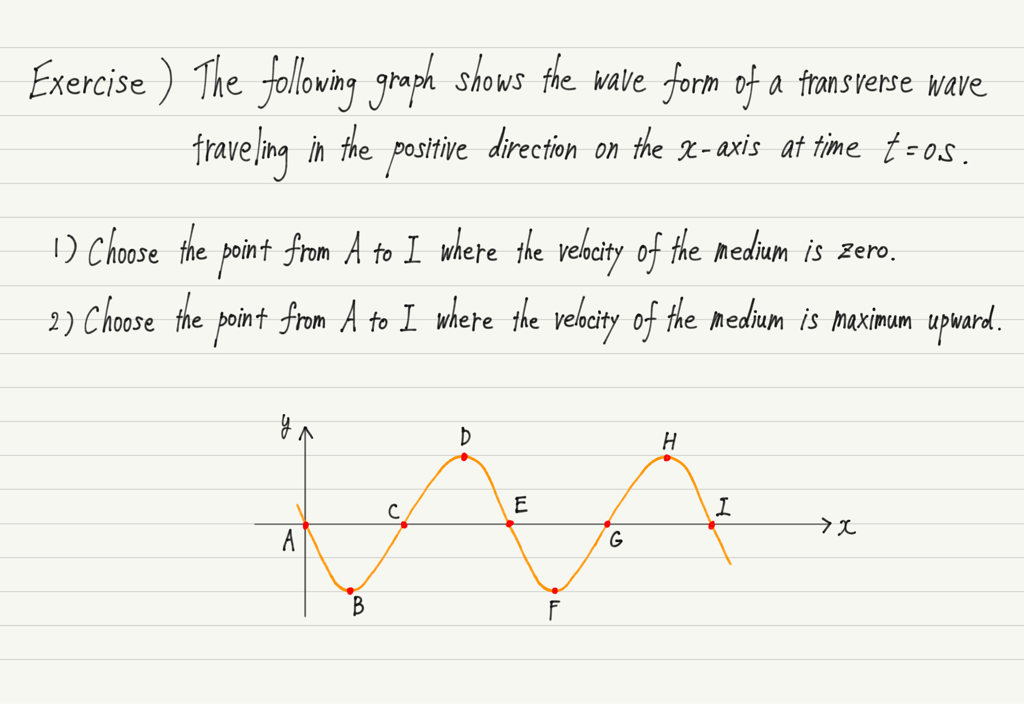Velocity of Medium

In previous lectures on reading graphs of waves, the main focus was on reading numerical values such as amplitude and wavelength.

Related articles

How to Read Wave Graphs

This time, instead of numerical values, let us read the motion of the medium.

Exercise

This question does not ask for numerical values, so it is difficult to know what to pay attention to in the graph.
Let’s get right to the explanation!

“Medium’s” velocity

First, to those who misunderstand the question without reading it carefully.
It is the “medium’s” velocity that is being questioned in this exercise, not the speed at which the wave is traveling!

Waves move at constant velocity (unless the medium changes), so there is no place where the velocity is zero.

Since we are being asked about the velocity of the medium, let’s focus on the motion of the medium, not the motion of the wave.

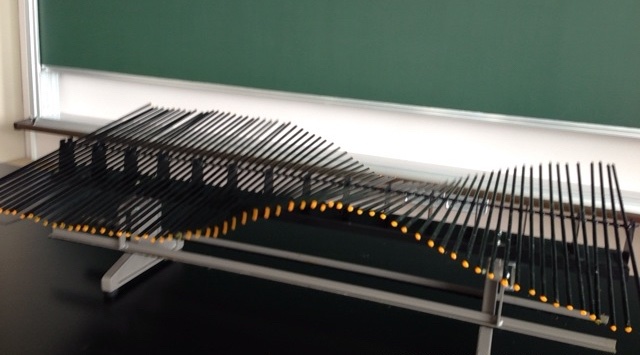
The usual example of a wave traveling down a rope is a good one, but the wave machine is probably the easiest way to understand the motion of the medium.

If you have seen it in school, remember the movement.
If you’ve never seen it before, you can watch it on YouTube.

Let’s start with 1). The medium of the wave machine is each bar in a line.
In other words, for this question, all we need to focus on is “the movement of the bars of the wave machine”!

When you look at a wave machine, you tend to look at the whole movement, but please be patient and follow only the movement of one bar.

Then you will notice that the bars are only going up and down and back and forth.
Once you realize this, you have already solved the question.

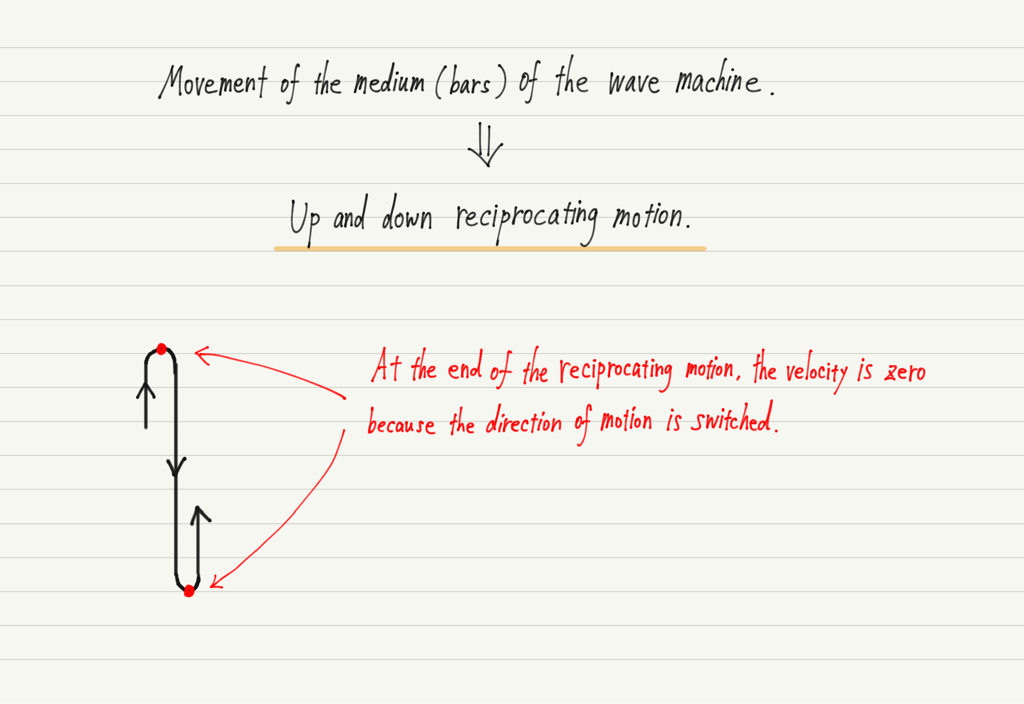
The point in the reciprocating motion where the velocity goes to zero is fixed at the top and the bottom!

Therefore, the answer to 1) is the top (=crest) and bottom (=trough) of the wave.
The answers are B, D, F, and H!

Direction in which the medium moves

Next 2). Now we look for the location where the upward velocity is maximum.
We have just seen that the medium’s velocity is zero at the top and bottom.

This means that “the medium decelerates as it moves upward and comes to rest at the highest point. And if it goes down, it slows down and comes to rest at the lowest point.”

If it slows down going up and down, where is it moving the fastest?

That is, of course, right in the middle!

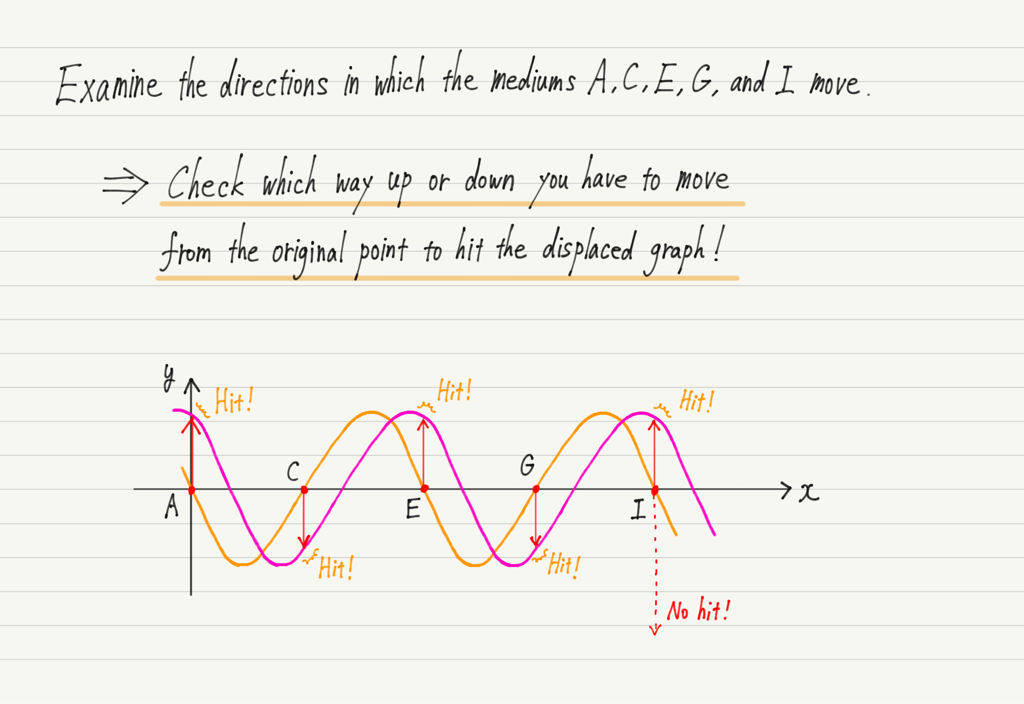
Therefore, the points of maximum velocity are the intersections with the x-axis, A, C, E, G, and I.

We are not done yet.
Since the question is “the point where the upward velocity is maximum,” we must also consider the direction.

The direction of the wave is also read from the graph, but since the y-x graph is a graph of a moving wave stopped in time (i.e., a “picture” of the wave), there is no way to tell how the medium is moving just from this graph.

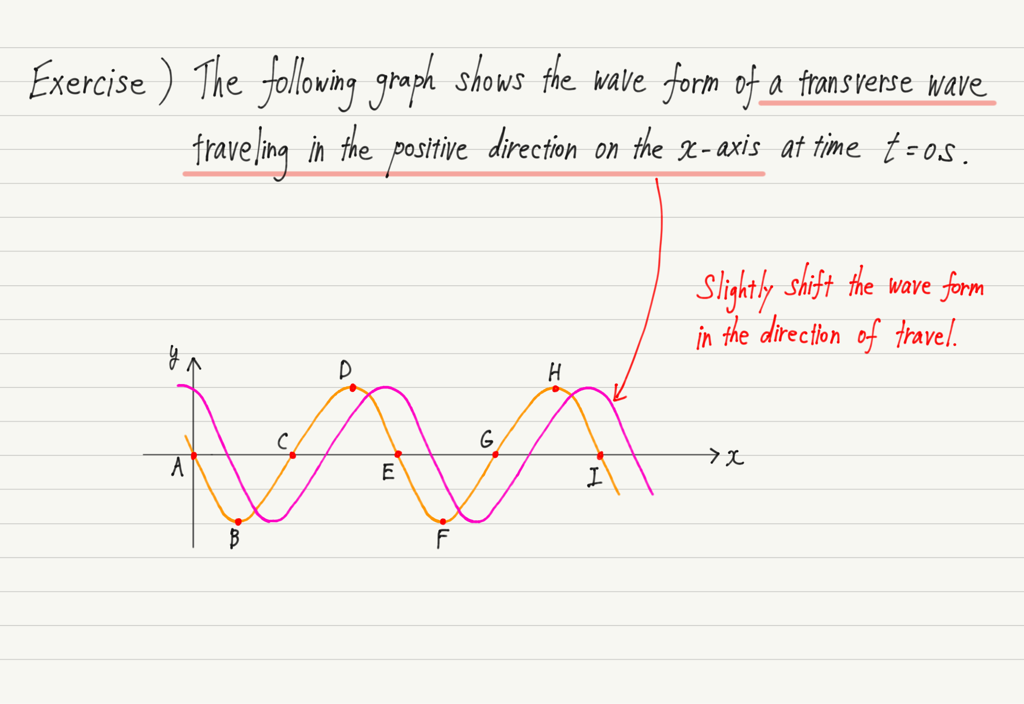
The solution is to “take a picture of the next moment and compare!”

Since a photograph is a metaphor, we are specifically writing a y-x graph for the next instant.
Since the statement in question gives the direction in which the wave is traveling, let’s shift the wave a little in this direction!

The shifted graph is the waveform at the instant following the time of the first graph.

Now, think of this graph as representing the waves of a wave machine.
The original waveform moves, resulting in a shifted waveform.
What will be the movement of the bars at A, C, E, G, and I ?

Remember that the bars of the wave machine can only move up and down.

Now you know whether each bar moved up or down!

The answer is the one with the arrow pointing up among A, C, E, G, and I.
That is, A, E, I !

In the exercise, we dealt with transverse waves, but the graph of a longitudinal wave can be considered in exactly the same way.
Note the direction of displacement for longitudinal wave graphs.

If the previous exercise is a longitudinal wave, then the answer to 2) represents “the point at which the velocity of the medium is at a maximum in the right direction”.
(Note that the right direction of the longitudinal wave is upward when it is plotted on the graph.)

Some wave questions, such as this one, cannot be solved without a firm understanding of the motion of the medium.
Always be able to picture in your mind the specific movements of the medium, be it a rope, a wave machine, or whatever!